Stress-element and plane stress
General stress state at a point:
At
each point in a loaded material body the stress can be characterized by a
stress element which shows the shear and normal stresses on a cube. The figure
shows such an element taken from a body under three dimensional loading.
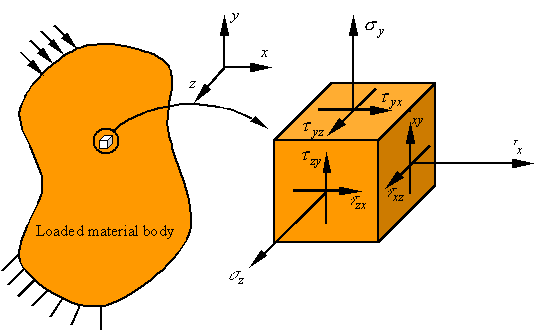
The first subscript on the shear stress denotes the normal
to the surface on which the shear stress is applied, and the second index
refers to the direction of the shear stress. It can be shown that the “stress
is symmetric,” meaning that

Plane stress:
The
state of stress referred to as plane stress is characterized by one surface
being free of traction. Taking the surface with normal along the z-direction
to be the free surface, the state of stress for plane stress can be drawn as
follows.
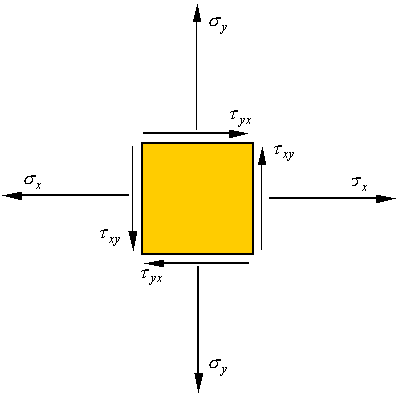
Stress on an inclined surface in
stress:
Consider
the plane stress element shown. If a section from this element is separated as
shown, the normal and shear stress on this surface can be calculated by the
following process.
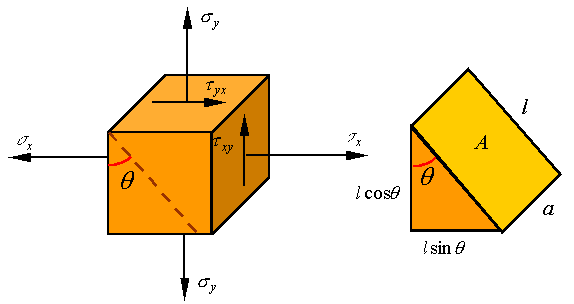
If
the area of the surface exposed by the cut is A, then the area of the
surface to the left will be ![]() and the area of the
surface at the bottom will be
and the area of the
surface at the bottom will be ![]() . The stresses on these surfaces are shown on the left below,
and the load on the surfaces (stress times area) are shown on the right below.
. The stresses on these surfaces are shown on the left below,
and the load on the surfaces (stress times area) are shown on the right below.
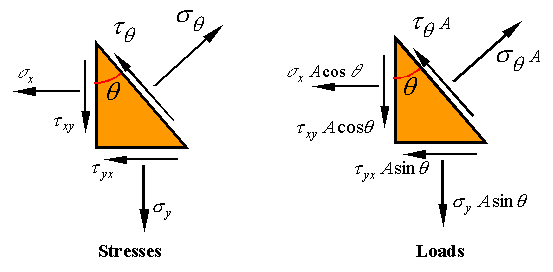
Equilibrium
of forces in the direction normal to the inclined surface requires that
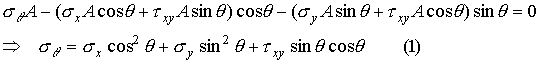
where
we have used the fact that stress is symmetric to replace ![]() by
by ![]() . Equilibrium in the direction tangent to the inclined
surface requires that
. Equilibrium in the direction tangent to the inclined
surface requires that

One
can use the following trig identities to reorganize these expressions.
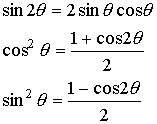
The
result of using these identities is
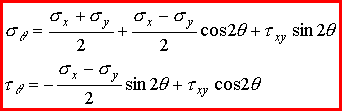
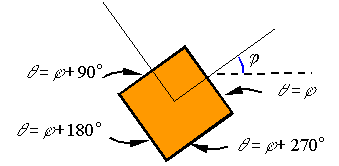
Elements selected along different coordinate directions:
If
in place of the x-y coordinate system the stress element is
oriented along the x*-y* coordinate
directions, the normal and shear stresses on the element will look as follows.
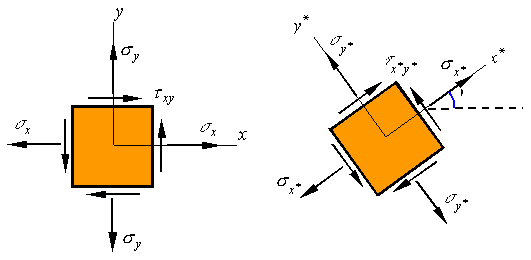
This
new coordinate system is rotated an angle ![]() counter-clockwise
from the original system. Each surface of this new element is an inclined
surface for which one can calculate the stresses using the above equations.
Note that the orientation of each of the four surfaces are given below.
counter-clockwise
from the original system. Each surface of this new element is an inclined
surface for which one can calculate the stresses using the above equations.
Note that the orientation of each of the four surfaces are given below.
Substitution
of these angles into the equations for normal and shear stress on inclined
surfaces results in
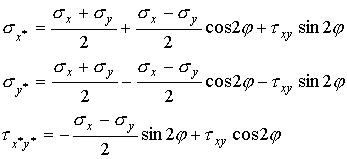
Note that the sum of the two normal stresses results in the relation
![]()
which states that the sum of the two normal stresses on a stress element should be the same irrespective of the coordinate directions selected.
![]()
ă Mehrdad Negahban and the University of Nebraska, 1996-2000.
All rights reserved
Copy and distribute freely for personal use only
Department of Engineering Mechanics, University of Nebraska, Lincoln, NE 68588-0526
Last modified at: 9:36
AM,
Wednesday, March 08, 2000